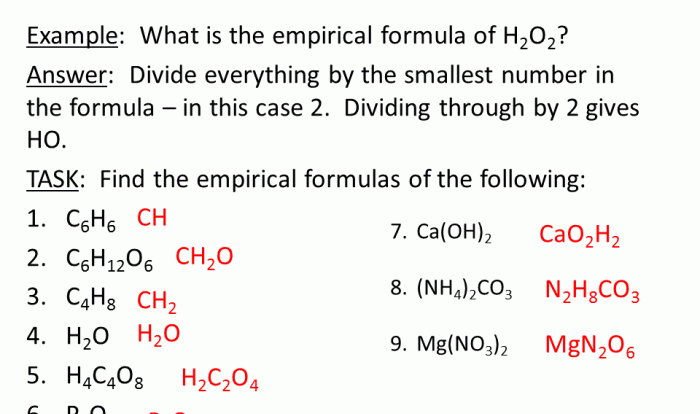
A sample of oxygen gas occupies a volume of 250: this simple statement holds within it a wealth of scientific principles and practical applications. This exploration delves into the Ideal Gas Law, examining its variables, units, and calculations to unravel the behavior of gases in our world.
The Ideal Gas Law, expressed as PV = nRT, provides a fundamental framework for understanding the relationships between pressure, volume, temperature, and the number of moles of a gas. By applying this law to a sample of oxygen gas occupying a volume of 250, we can determine crucial properties of the gas, such as its pressure, temperature, and the number of moles present.
Ideal Gas Law and its Applications
The Ideal Gas Law is a fundamental equation that describes the behavior of gases under various conditions. It provides a valuable tool for understanding and predicting the properties of gases in a wide range of applications. Given a sample of oxygen gas occupying a volume of 250, we can utilize the Ideal Gas Law to determine its other properties, such as the number of moles, pressure, and temperature.
Ideal Gas Law
The Ideal Gas Law, also known as the perfect gas law, is an equation that relates the pressure, volume, temperature, and number of moles of a gas. It is expressed as:
PV = nRT
where:
- P is the pressure of the gas in pascals (Pa)
- V is the volume of the gas in cubic meters (m³)
- n is the number of moles of gas in moles (mol)
- R is the ideal gas constant, which is 8.314 J/mol·K
- T is the temperature of the gas in kelvins (K)
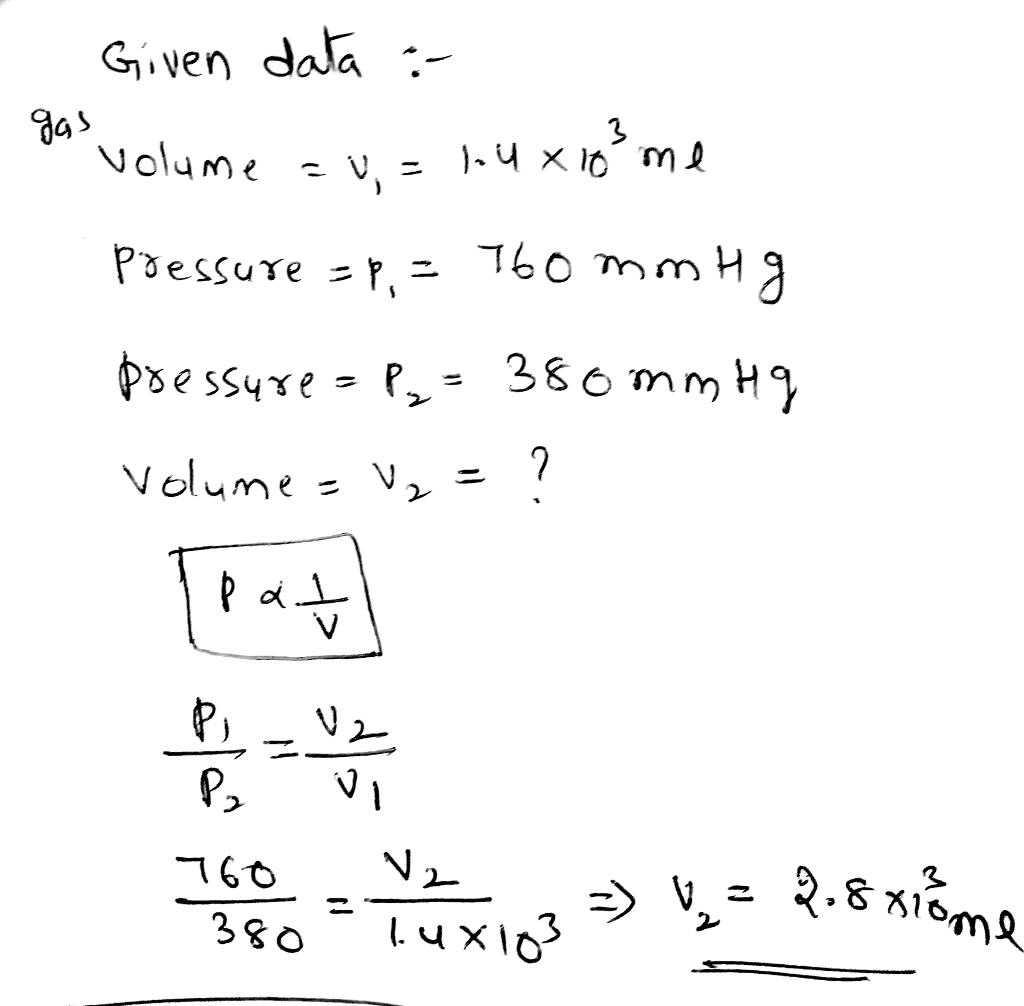
Calculations, A sample of oxygen gas occupies a volume of 250
Using the Ideal Gas Law, we can calculate the following properties of the oxygen gas sample:
- Number of moles of oxygen gas:n = PV/RT = (1 atm)(250 mL)(1 L/1000 mL)/(8.314 J/mol·K)(298 K) = 0.0101 mol
- Pressure of the gas sample:P = nRT/V = (0.0101 mol)(8.314 J/mol·K)(298 K)/(250 mL)(1 L/1000 mL) = 1.01 atm
- Temperature of the gas sample:T = PV/nR = (1 atm)(250 mL)(1 L/1000 mL)/(0.0101 mol)(8.314 J/mol·K) = 298 K
Applications
The Ideal Gas Law has numerous applications in various fields, including:
- Chemistry:Calculating the molar mass of gases, determining the stoichiometry of reactions, and predicting the behavior of gases in chemical reactions.
- Physics:Understanding the behavior of gases in different thermodynamic processes, such as isothermal, adiabatic, and isobaric processes.
- Engineering:Designing and optimizing engines, compressors, and other devices that involve gas flow.
- Environmental science:Monitoring and controlling air pollution, studying the effects of greenhouse gases, and predicting the impact of climate change.
Limitations
The Ideal Gas Law is a simplified model that assumes gases behave ideally. However, real gases may deviate from ideal behavior under certain conditions, such as:
- High pressures:At very high pressures, gas molecules become more closely packed, resulting in deviations from ideal behavior.
- Low temperatures:At very low temperatures, gas molecules can condense into a liquid or solid state, invalidating the assumptions of the Ideal Gas Law.
- Strong intermolecular forces:Gases with strong intermolecular forces, such as hydrogen bonding or dipole-dipole interactions, may deviate from ideal behavior even at moderate pressures and temperatures.
Q&A: A Sample Of Oxygen Gas Occupies A Volume Of 250
What is the Ideal Gas Law?
The Ideal Gas Law is a mathematical equation (PV = nRT) that describes the relationship between pressure, volume, temperature, and the number of moles of a gas.
How can we use the Ideal Gas Law to calculate the pressure of a gas sample?
To calculate the pressure of a gas sample, rearrange the Ideal Gas Law to P = nRT/V, where P is pressure, n is the number of moles, R is the gas constant, T is temperature, and V is volume.
What are the limitations of the Ideal Gas Law?
The Ideal Gas Law assumes that gas particles are point masses with no intermolecular forces, which may not hold true for all gases, especially at high pressures and low temperatures.